Apply Now
Effective Ways to Calculate Margin of Error in 2025
Understanding Margin of Error: Definition and Importance
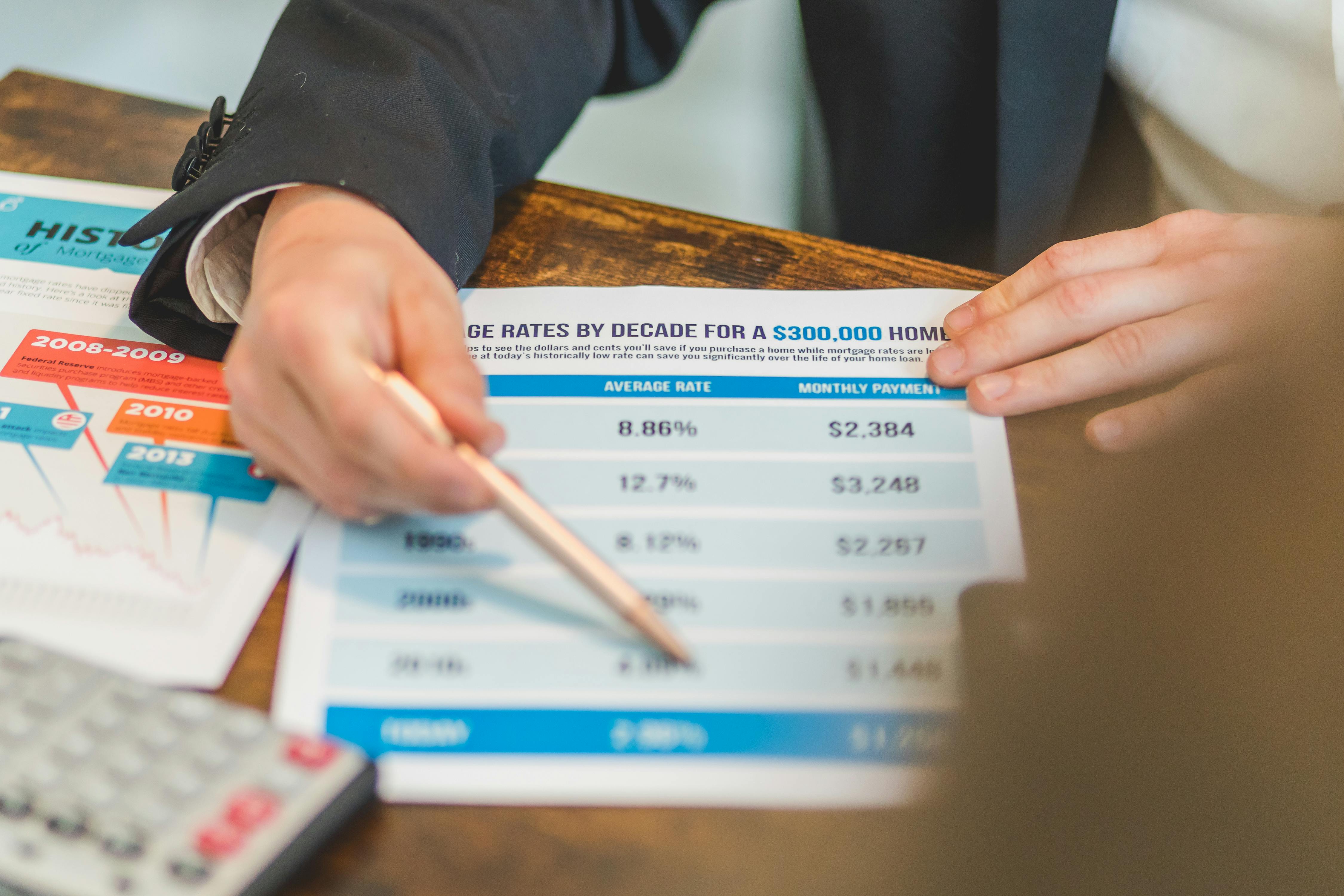
The concept of **margin of error** is critical in statistics, particularly in research and polling. Defined as the amount of random sampling error in a survey's results, it provides a range within which the true value in the population is expected to fall. For instance, if a survey indicates that 60% of respondents prefer a particular product, with a margin of error of ±3%, it suggests that the actual percentage in the population could be anywhere between 57% and 63%. Understanding this fundamental statistic helps clarify the **implications of margin of error** for decision-making and public opinion analysis.
The **significance of margin of error** becomes even more apparent when considering how data interpretation can influence business and policy decisions. A small margin of error indicates a higher level of confidence in the results, while a larger margin suggests uncertainty. This is crucial in fields like market research and public opinion polling, where precise estimations can drive key strategies.
Honing in on how to **compute margin of error** effectively establishes reliability in your statistical analysis. The formula for the **margin of error** typically involves the sample size and the confidence level selected for the research, which reflects how confident you want to be about the results.
This foundational understanding leads us to a deeper exploration of the various methods of calculating the margin of error.
Calculating the Margin of Error: The Essential Formula
To **calculate margin of error**, one must first determine the appropriate formula based on the context. Generally, for a simple random sample, the formula is:
\[ \text{Margin of Error} = Z \times \frac{\sigma}{\sqrt{n}} \]
Where:
- \(Z\) is the Z-score representing the desired confidence level (e.g., 1.96 for 95% confidence),
- \(\sigma\) is the standard deviation of the population (or an estimate from the sample),
- \(n\) is the sample size.
For proportions, the formula slightly alters to:
\[ \text{Margin of Error} = Z \times \sqrt{\frac{p(1-p)}{n}} \]
Where \(p\) represents the sample proportion.
This section encapsulates how to use statistical software or calculators to streamline this process, enhancing efficiency in **error margin calculation techniques**. For instance, programs like R and Python can automate these calculations on large datasets, providing timely and accurate results to researchers.
Real-world implications of **calculating error margins** accurately cannot be overstated. As businesses engage more with data-driven strategies, these calculations can mean the difference between successful product launches and costly failures. Properly applying the margin of error formula in different types of studies, including qualitative and quantitative research, strengthens the overall research methodology and ensures reliability in findings.
Building on the mathematical framework, we need to understand how sample size impacts margin of error.
Influence of Sample Size on Margin of Error
The sample size plays a pivotal role in determining the **margin of error**. Generally, the larger the sample, the smaller the margin of error, leading to more accurate population estimates. This relationship is rooted in the law of large numbers, which states that as the sample size increases, the sample mean will converge closer to the population mean.
To illustrate, if a researcher uses a sample of 100 respondents, they might find a margin of error of ±5%. However, employing a sample of 400 respondents could reduce that margin to ±2.5%. Therefore, understanding the **population margin of error** versus **sample margin of error** helps researchers make informed decisions regarding their studies.
Practical applications such as in surveys reveal that increasing the sample size not only improves estimation accuracy but also enhances the overall **data accuracy** and **research reliability**. However, it’s crucial to balance sample sizes with budget constraints and logistical considerations; excessively large sample sizes can lead to diminishing returns regarding the accuracy gained versus resources used.
Furthermore, researchers should be aware of common mistakes: assuming larger samples always equate to fewer errors without considering the survey's design can lead to interpretative issues. Evaluating the costs associated with increasing sample sizes is also an essential aspect of comprehensive **margin of error analysis**.
Next, we explore effective strategies for **reducing margin of error** in practical survey applications.
Strategies for Reducing Margin of Error
Reducing the margin of error can significantly enhance the reliability of research findings. Below are several **effective ways to adjust margin of error**:
1. **Increase Sample Size**: As discussed, a larger sample leads to a smaller margin of error. Balancing the increased cost and time investment is vital in this approach.
2. **Enhance Data Quality**: Ensuring accurate and reliable data collection methods reduces variability and improves estimation quality, thus lowering the margin.
3. **Use Stratified Sampling**: This approach involves dividing the population into subgroups and sampling from these groups to ensure representation, thereby refining the estimate and reducing error margins.
4. **Adjust Confidence Levels**: Consider whether your confidence level is set too high for the type of research undertaken. Lowering this level slightly can lead to a smaller margin of error at the expense of certainty.
5. **Utilize Technology**: Online survey tools often include built-in features to calculate and recommend optimal sample sizes based on user-defined confidence levels and margins of error.
Learning about these methods allows researchers to make informed choices when designing studies, foreseeing **implications of statistical error**, and enhancing overall trust in their results. Transitioning into practical implementations, we look at how margin of error is presented in real-world scenarios and surveys.
Its part of generated content. Can i generate another part?